Problem of the Week: An unknown function with two variables [Algebra]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.

The Fibonacci Sequence and Golden Ratio in Nature!

You have probably heard of Fibonacci's famous sequence: 1, 1, 2, 3, 5, 8..., and so on, with each term equalling the sum of the previous two terms. If you divide each term by the previous result, you will find that as the numbers get bigger, the ratio converges to 1.6182. 1.6182:1 is known as the 'golden ratio'. This seemingly random sequence has baffled mathematicians and scientists alike, as the sequence and its converging ratio keep appearing in nature. Here are just a few places they appear in nature:

Flowers

The Fibonacci sequence can be seen in two different places in flowers. The most visible place is the petals of a flower. Several flowers have petals that are numbers of the fibonacci sequence. Lilies have 3 petals, buttercups have 5 petals, and daisies have 34 petals, for example. Scientists have theorized that petals that fit the Fibonacci sequence absorb more sunlight, among other uses.

Additionally, the seeds a flower produces follow the Fibonacci sequence. The seeds bunch up in the middle and spiral out in the same shape as a Fibonacci spiral. 

Shells

The spiral shaped Nautilus shell perhaps most closely resembles the Fibonacci spiral. Scientists speculate that the reason shells tend to be shaped like the Fibonacci spiral is due to the natural order taking the most efficient route as possible to create a spiral. Below is a comparison between the spiral and shell.

Galaxies

Galaxies maintain the shape of a Fibonacci spiral, seemingly against Newtonian principles! Because the stars have differing angular velocities depending on their distance from the center of the galaxy, the spirals shouldn't remain intact. However, the stars on the outside move faster than expected, allowing the galaxies to maintain the spiral shapes!

And finally...

Cats!

PotW: Solving for the sum of x + 2 and x - 2 to the -0.5 power [Algebra]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.

NBA Stats to Pay Attention to

The NBA season tipped off last night, so it's safe to say that basketball is back! The games themselves will be incredible, but at the center of math, we think that the statistics are pretty interesting as well. We know the basic stats - points, assists, rebounds, etc. - but what about the advanced stats? Here's a quick peek at advanced stats in the NBA - and what they measure!

Player Efficiency Rating (PER)

This stat was created by John Hollinger, a basketball analyst, as a statistic that measures how efficient a player is. It is often confused as a stat that measures everything about a player, but even Hollinger has admitted that it can't do this. What this statistic essentially does is take into account how many good things a player does (points, assists, rebounds, blocks, steals, field goals made, etc.), subtracts the bad things a player does (turnovers, missed field goals, etc.), and take into account the number of possessions used by a player (as well as how fast or slow their team plays) to arrive at one number. The statistic tends to undervalue good defenders, because while blocks and steals offer some measure of how good a player defends, it certainly doesn't measure how good a defender is.  The complete calculations can be found here. The average player is supposed to have a PER of 15. The league leader last year was Giannis Antetokounmpo (above), the league MVP, who has a PER of 30.89. The best season ever was Wilt Chamberlain in 1962-63, when his PER was 31.82.

Win Shares

Like PER, Win Shares is a statistic that tends to figure out how efficient a player is. However, unlike PER, Win Shares take playing time into account. Let's say a player plays two minutes, and in this time scores two points and gets a rebound. This is a remarkably efficient line, and PER would reflect this by rewarding the player with a high number. However, the numbers would be essentially unsustainable if taken over a longer period of time (the numbers extrapolated over 36 minutes, roughly what an NBA starter plays per game, are 36 points and 18 rebounds per game, totals only reached by the same player in one season by Wilt Chamberlain). Win Shares, on the other hand, would reward this efficiency and small sample size, but not too much. It rewards efficiency, but also quantity of time played. The calculations behind Win Shares can be found here. The leader in Win Shares last year was James Harden (above) with 15.2 Win Shares. The best season all time for Win Shares was by Kareem Abdul-Jabbar in 1971-72, with 25.37 Win Shares. 

True Shooting Percentage (TS%)

Steph Curry is considered to be one of the best shooters ever. However, last season, he ranked 57th in the league in field goal percentage. Why is that? The further away from the hoop, the harder it is to score. (This is why people win thousands of dollars for half court shots, but rarely do so for layups) Curry tends to shoot the ball further away from the hoop - namely three pointers, a shot that is more valuable than a two point shot. True shooting percentage takes into account all types of shots (two pointers, three pointers, and free throws) and weights them to arrive at a number that is a more accurate measure of how good a shooter a player is. While Curry ranked 57th in the league in shooting percentage last year, he ranked 7th in TS%. The formula for calculating TS% can be found here. The leader in TS% last year was Rudy Gobert (above) with .682. The all time best season in TS% was .7082, done in 2011-12 by Tyson Chandler.

Box Plus-Minus (BPM)

Plus-Minus is a fairly elementary stat. It simply states how much better the team did with a player on the floor. If Lebron James enters a game tied 50-50 and leaves with his team leading 67-62, his plus minus is equal to +5. However, this stat can be misleading as well. It would be far harder to score on an all star team than against the back ups of the worst team in the league. BPM takes this into account, resulting in a statistic that measures how good a player would be when paired with 4 average players against 5 average players. The methodology behind it can be found here. The leader in BPM last year was James Harden with a BPM of +11.7. The all time best season in BPM was from Russell Westbrook (above) in 2016-17, which was +15.6.

Problem of the Week: Summing Dice [Probability]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.

Will the Millennium problems ever be solved?

Given that it is the spookiest time of the year, we felt that there is no topic more appropriate to talk about today than the Millennium Problems: the world's most daunting mathematics problems!

What are the Millennium Problems?

The Millennium Problems are seven mathematic and computer science problems presented by the Clay Mathematics Institute in 2000. The Institute offers a one million dollar prize to anyone who solves them. The famous seven problems are:

1. Yang-Mills and Mass Gap
2. Riemann Hypothesis
3. P vs NP Problem
4. Navier-Stokes Equation
5. Hodge Conjecture
6. Poincaré Conjecture
7. Birch and Swinnerton-Dyer Conjecture

How many of them been solved?

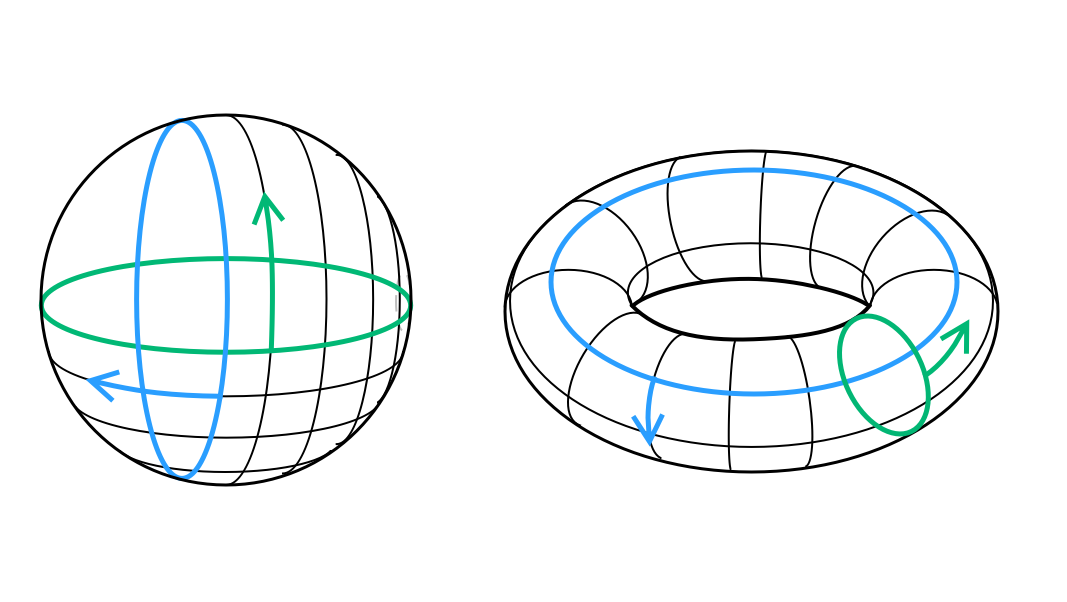
So far, only one of the seven problems has been solved. In 2003, Russian mathematician Gregori Perelman solved the Poincaré Conjecture - building off of work from Richard S. Hamilton and a century of work from mathematicians trying to prove it. Perelman was offered the Fields Medal, but declined it.

How close to being solved are the unsolved problems?

Over the last nineteen years, dozens of solutions have been submitted for these problems. Many of them have not held up to peer-review, and some of them are currently being peer-reviewed. The late British mathematician Michael Atiyah submitted a solution to the Riemann Hypothesis in 2018, which was met with both excitement and skepticism.

For more information about the millennium problems, check out the CMI website!

Problem of the Week: Solving for x and y with variables in the denominator [Algebra]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.

Monster Math: Vampire Numbers!

Now that it's October, the Worldwide Center of Mathematics will be dedicating Thursdays to Monster Math! While math is a little scary at times and monsters are always spooky during October, this post will be fright free, and we hope you'll learn something new!

Today's Spooky Subject is: Vampire Numbers!

What is a Vampire Number?

A vampire number is a number with even digits (greater than two digits) such that there exist two factors, each with half the digits of the original number with at least one of them not having a trailing zero, such that the digits of the two factors combine to be the same as the original number. For 1530 has the factors, or 'fangs', of 51 and 30. Because 51 and 30 have a product of 1530 and their digits can be rearranged to 1530, 1530 is a Vampire Number!

There are 10 vampire numbers with 4 digits: 1260 (21 and 60), 1395 (15 and 93), 1435 (41 and 35), 1530 (51, 30),  1827 (21 and 87), 2187 (81 and 27), and 6880 (86 and 80).

Some vampire numbers have multiple pairs 'fangs.' For example, 125460=204*615=246*510.

Vampire numbers with 3, 4, and even 5 pairs of fangs have been found. The smallest vampire number with 5 pairs of fangs is 24959017348650! 

Are there other kinds of Vampire Numbers?

Unlike Vampire Numbers, whose fangs must have the same number of digits, Pseudovampire Numbers do not need fangs with the same number of digits. In addition, a Pseudovampire number can have more than two fangs. Examples of pseudovampire numbers include 126 (6*21) and 1395 (5*9*31).

Prime Vampire Numbers are Vampire Numbers with prime fangs. The smallest Prime Vampire Number is 117067 (701*167).

Finally, the Roman Numeral Vampire Number is exactly what it sounds like: a Vampire Number that exists with Roman Numerals! While 8 isn't a vampire number, using the Roman Numeral system, we find that it is a Vampire Number! II*IV=VIII, making 8 a Roman Numeral Vampire Number!

Problem of the Week: Probability of an Obtuse Triangle [Probability]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.