Four Mathematical Artists!

To quote Danica McKellar, "One of the most amazing things about mathematics is the people who do math aren't usually interested in application, because mathematics itself is truly a beautiful art form. It's structures and patterns, and that's what we love, and that's what we get off on." Mathematics and art have always been linked. Studying mathematics requires a creativity that resembles art, and artists often borrow concepts from mathematics in their art. Here are a few artists who have been inspired by mathematics in their works!

MC Escher

Relativity by MC Escher (Source: Vogue Australia)

Escher was interested in several fields of mathematics, including both Euclidean and non-Euclidean geometry and topology. He explored several of these ideas in his pieces. Tessellations and Mobius strips are among his mathematical explorations. Although he never considered himself a mathematician, he was well-regarded by and mingled with mathematicians at the time, and even conducted research into tessellations!

Leonardo Da Vinci

Vitruvian Man by Leonardo Da Vinci (Source: WikiArt)

The original Renaissance Man, Da Vinci is most well known for the Mona Lisa. However, his interest in math and science appeared in his art and many of his sketches. The Vitruvian Man (pictured above) shows what Da Vinci believed to be the geometrically ideal man. Additionally, he used mathematical concepts such as the golden ratio and played with linear perspective in The Last Supper and the Mona Lisa!

The Writers of Futurama

From "The Prisoner of Benda" by Ken Keeler (Source: Buzzfeed)

We have talked about Futurama and math before on this blog and writing may not technically be art, but several of the writers of this cartoon were well versed in mathematics. Several of them, including the show's creator David X. Cohen, published papers in mathematics, and mathematical concepts have been presented on the show, including a theorem created for the show!

Ada Dietz


From Algebraic Expressions of Handwoven Textiles by Ada Dietz (Source: Flickr)

Dietz was a math teacher who decided to apply what she taught in her crafts! Her best known work is the monograph titled Algebraic Expressions of Handwoven Textiles, which is an instructional book showing how to incorporate algebra into woven crafts. To learn more about her methods and to see her designs check out this site !

Problem of the Week: Subsets with no pairs summing to 11 [Combinatorics]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.

Mathematicians in the Movies

The math that many mathematicians pioneers captures the imagination, piquing the interest of millions. Some of their life stories have been equally captivating, and Hollywood has often used them as the subjects of biopics, especially during awards season. If you're looking for a movie about math to watch, try one of these out!

(All images from Wikipedia)


The Man Who Knew Infinity


Starring Dev Patel as the prodigal Indian mathematician Srinivasa Ramanujan, the movie tells his incredible life story from living in poverty to studying at the University of Cambridge. A remarkable mathematician with a life worthy of the big screen, Ramanujan is responsible for ideas such as the Ramanujan Prime and partition formulae.

A Beautiful Mind


This movie about economist John Nash earned 8 Oscar nominations and 4 wins, including for Best Picture! Starring Russell Crowe as Nash in an Oscar nominated performance, the film shows how the economist dealt with schizophrenia in the early stages of his academic career. The only winner of the Nobel Prize and the Abel Prize, Nash's works include studying game theory and nonlinear partial differential equations.

The Imitation Game


Set during World War II and portraying the efforts to crack the enigma, this movie received eight Oscar nominations. Starring Benedict Cumberbatch as Alan Turing and Keira Knightley as Joan Clarke. Along with depicting the British scientists and code breakers, the film also focuses on how Alan Turing was punished by the British government due to his homosexuality.

Hidden Figures


(Clockwise from top right: Johnson, Jackson, Vaughan)


A lot of hard working mathematicians worked to get a man on the moon, but few were as discriminated on their mathematical journeys as three black, female scientists at NASA. Starring Taraji P Henson, Octavia Spencer, and Janelle Monae as, respectively, Katherine G. Johnson, Dorothy Vaughan, and Mary Jackson, this movie depicts the perseverance they showed in the face of adversity and the contributions they made to science and mathematics.

Theory of Everything


Taking place during Stephen Hawking's years as a graduate student at the University of Cambridge, the film portrays Hawking struggling through motor neuron disease as he begins his long career studying black holes and the universe. Eddie Redmayne won an Oscar for his portrayal of the late astrophysicist, and the film itself was nominated for 5 Oscars in total.

Problem of the Week: Finding the sides of a Square [Geometry]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.

Advice for an Undergraduate Math Major (from an Undergraduate Math Major)

It's that time of year. Back to school ads are popping up. Pencils and calculators are being purchased. Syllabi are being sent out. Whether you're an incoming freshman or a senior with an eye out the door, each year brings all the stress of college life. There's no book on how to have a perfect college experience without some troubles, but there are a few things to try as you enter the new school year!

Do Practice Problems!
No one will be hovering over your shoulder making sure you understand all the math being taught to you. Lectures will cover the material, but there won't be a lot of examples assigned to you. The best way to learn the material will be to find problems to do. Sometimes professors will have graded homework, but many of them will expect you to do problems by yourselves. They will usually tell you which problems they want you to try so you can learn the material. There will be an occasion (or two, or three, or ... ) when you don't understand a problem or how to do the math. What should you do then?

Use your computer!
Sometimes, the best way to figure out a concept is to watch someone do the problem out step by step. Or to watch someone do as many problems as it takes until the concept makes sense. The easiest and most convenient place to find problems done out is, of course, the internet. Whether you want solutions to problems done out, or want a broad subject explained in depth, there are plenty of resources online. WCoM, for example, offers videos ranging from exploring the basics of different subjects within mathematics to doing out solutions of practice problems found in textbooks.                                                                                                                                                                                 
Go to Office Hours! 
Professors can seem intimidating. They have been studying mathematics for years and years, so it can be scary to approach them with a problem they've been teaching for a long time. However, every professor will at the very least give you the help necessary to understand the material, which is a very good minimum. The majority of math professors, however, will be able to offer much more. They can be references for job applications. They can offer research opportunities. And they are also a resource for any questions about math that you may have. Want to know about what kind of careers you can do with a math degree? Curious about a field of mathematics? There is no better resource for any sort of question you have about mathematics than office hours. And if you can't make office hours, many professors will be accommodating to your schedule and will be able to find time to meet with you.

Work with Friends!
Fortunately (or unfortunately, depending on your viewpoint) math is a subject with not a lot of group projects. This can make it easy to isolate yourself when doing your work. However, to quote a professor who told me this (during office hours!), math is a social activity, and should be treated as such. This isn't to say that you should copy your friend's work or offer your own work to be copied. Working together on a problem will ensure that both you and your friend understand the material and get the problem right. I've tried to take on many homework assignments by myself and ran into many hurdles along the way. Hours have been spent trying to figure out what I'm doing wrong. However, after 5 minutes with a friend, these hurdles are usually cleared. Two minds are better than one, and no where is that more true than on a math problem you can't seem to solve.

Take Breaks!

The college workload will be a lot, and there will be pressure to study all night and all day sometimes. However, there is a lot of value in taking a step away from work for a little bit. Taking a break to work out, take a walk outside, or spend time with friends is usually a good way to recover from all the work. After the break, problems that you've stared at for hours on end start to look a little more clear.

Get some sleep!
Everyone knows the stereotypical college student camped out in the library, cans of Red Bull scattered around them, pulling an all nighter studying. While that might seem like the best way to teach yourself multivariable calculus, you shouldn't ever sacrifice sleep in order to learn! The best way to go about learning something is to do a little at a time rather than all at once before a big test. I certainly can't say that I haven't crammed before, but the best test grades are those that come from taking time to learn the material and understand the concepts, while getting as much sleep as possible.

We hope this advice helps! And remember that the Center of Math website has plenty of resources for Math majors!

Problem of the Week: Rolling 4 Dice [Probability]

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Solution below.

A Brief History of Zero

A Brief History of 0

Today, the existence of zero is a given. After finishing up some dessert, people say things like "there are zero cookies left" and "I have zero energy to get up" without thinking much about throwing zero into their sentence. (Presumably their minds are far more occupied on all the sugar they just ate) However, up until relatively recently in human history, zero as a concept was unheard of!

All across the world, people thought of number systems soon after they needed to keep track of how much stuff they had. If you have five sheep and give one away, it is helpful to write down that you now have four sheep so you won't frantically wonder where the missing sheep is. Number systems popped up from India to Greece to Rome to Central America. However, these number systems were usually only limited to positive integers. There was no need to account for negative livestock!

The first use of zero came about in a few number systems as placeholders. This didn't occur in all number systems. The Roman system didn't need placeholders; they used letters to identify a given quantity, and a letter was added to determine differences in quantity. However, the Arabic number system, for example, needed placeholders in order to determine the difference between numbers like 404 and 44. Without the 0 indicating that there are no tens in 404, 404 and 44 would be indistinguishable. While the Mayan number system developed this independently, the adoption of zero as a placeholder throughout much of Asia came about through trade routes.

Up until the 600s, the use of zero was still as a placeholder rather than a unique integer of its own right. However, a mathematician named Brahmagupta argued that zero should be treated as an integer, with all the properties of an integer - including the tricky situation of division. This idea gained traction throughout most of Asia, but failed to immediately make its mark in Europe, which still held on tightly to the Roman system of counting. Only in the 1200s, when European mathematicians such as Fibonacci advocated for the more useful Arabic numerical system, did Europe adopt zero as an integer and concept.

This adoption ended up proving useful a few hundred years later, during the time of Isaac Newton. As anyone who has taken calculus knows, the limit definition of a derivative requires dividing an expression by something that approaches zero. This fundamental idea of calculus
wouldn't have been possible without the idea that the absence of something should be something that should be written down, and then processed as an integer.

Problem of the Week: Solving for 2 unknown variables

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!

Video and written solution below!

4 Mathematical Paradoxes (and How to Solve Them!)

When people talk about math, usually what comes up is a rigidity and order to how things are supposed to go. However, mathematicians have for years uncovered and written about situations that don't make logical sense, and tried to figure out how they can be resolved. While there are thousands of paradoxes from A (Abelson's Paradox) to Z (Zeno's Paradox), this will only cover 4 paradoxes and their solutions. (for more paradoxes, explore this list on Wikipedia: https://en.wikipedia.org/wiki/List_of_paradoxes)

Without further ado, here they are!

1. Achilles and the Tortoise


















The great warrior Achilles is challenged to a race 100 meter long by a Tortoise. Knowing of Achilles' speed, the Tortoise asks for a 10 meter head start. Achilles tells the Tortoise that the head start will be nothing, as he can run 10 meters in a second. The Tortoise responds that, in the time it takes Achilles to run 10 meters, it will have run 1 meter. When Achilles runs that meter, the Tortoise will have run .1 meters. When Achilles runs .1 meters, the Tortoise will have run .01 meters. Thus, Achilles will seemingly never be able to catch the Tortoise despite being much faster.

Achilles, will of course, be able to catch the Tortoise. The paradox can be resolved by thinking about it one of two ways:
- The distance between them gets smaller by a factor of 10. The distance between the two will be decreased by a factor of 10 infinitely many times in theory, so the distance between them will eventually equal zero. At this point, Achilles and the tortoise will be next to each other, and Achilles will pass the Tortoise.
- Let Achilles' speed be 10 and the Tortoise's speed be 1. At time t, Achilles' position will be 10t and the Tortoise's will be t+10. These positions will be equal when 9t=10. After this time, Achilles will be ahead of the Tortoise. What will their position be? 11.11111...

2. The Boy or Girl Paradox



You're visiting a couple of statisticians who have two children. You know neither of the children's genders. After you knock on the door, a man arrives at the door, with his son poking his head around the corner. What is the probability that the other child is a boy as well, assuming that the probability of a child being a boy is 50% and being a girl is 50%?

It might seem fairly straightforward, given that there's a 50% probability of a child being a boy. However, we have to remember that there are 2 children total. View the problem from the perspective of knowing neither of the children's genders. There are 4 possibilities for the genders of the kids, with the oldest child having their gender listed first: MM, MF, FM, and FF. These probabilities are all equal. Having seen that one child is a boy, we can only eliminate the final option as a possibility. Thus, the three possibilities for the children are MM, MF, and FM. These all have equal probabilities, so the probability that the other child is a boy is equal to one third.


3. The Potato Paradox



Let's say you have a 100 pound bag of potatoes. 99% of the weight of potatoes is water. You decide to let your  bag of potatoes dehydrate until the weight of the bag is only 98% water. After doing this, you're astonished that your bag of potatoes now weighs 50 pounds!

How does making water a slightly smaller percentage of the weight change the weight so dramatically? We know that the original bag was 99% weight by water, so this means that 1 pound of the weight came from the 'solid' part of the potatoes. Thus, after dehydration, 1 pound of solid accounts for 2% of the weight of the bag. We can thus solve for x, where x is the weight of the bag, for 1 = .02x. Thus, x = 50 pounds.

4. The St. Petersburg Paradox



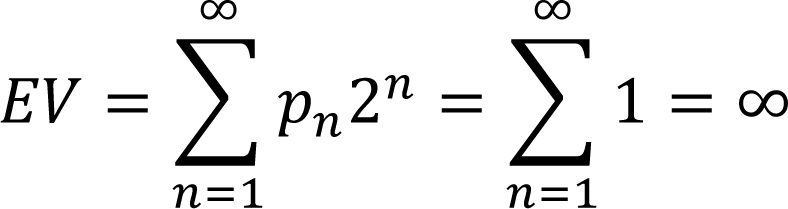
While you're walking one night, a friend approaches you with a fair coin and asks to play a game. They tell you that they will give you $2 if it lands on heads once, $4 if it lands on heads twice in a row, $8 if it lands on heads three times in a row, $16 if it lands on heads four times in a row, and so on and so forth. The game will end as soon as the coin lands on tails. After explaining the rules, your friend asks you if you want to wager $100 to play the game. Being a mathematician, you decide to calculate whether or not this would be reasonable. After doing the math, you quickly pull out a $100 bill and ask to play.

Why would you do this? The formula for expected value suggests that this is a smart idea. The formula for expected value is:
E = P(A)*A + P(B)*B +P(C)*C + ....
We plug in the probabilities of the coins landing on consecutive heads and the value you'll be given to find:
E= 0.5*2 + 0.25*4 + .125*8 + ... = 1 + 1 + 1 + 1 + ....
This value will be infinite. While at face value there doesn't appear to be a solution to resolve the paradox, economists and mathematicians have worked on an equation that gives a finite result to this equation - making it more in line with the thinking that one wouldn't bet everything on a game where earning everything back would be very unlikely!